Find the value of the expression if the unit vector of the vector. How to find the displacement module in physics? (Maybe there is some universal formula?)
There will also be problems for you to solve on your own, to which you can see the answers.
Vector concept
Before you learn everything about vectors and operations on them, get ready to solve a simple problem. There is a vector of your entrepreneurship and a vector of your innovative abilities. The vector of entrepreneurship leads you to Goal 1, and the vector of innovative abilities leads you to Goal 2. The rules of the game are such that you cannot move along the directions of these two vectors at once and achieve two goals at once. Vectors interact, or, speaking in mathematical language, some operation is performed on vectors. The result of this operation is the “Result” vector, which leads you to Goal 3.
Now tell me: the result of which operation on the vectors “Entrepreneurship” and “Innovative abilities” is the vector “Result”? If you can't tell right away, don't be discouraged. As you progress through this lesson, you will be able to answer this question.
As we have already seen above, the vector necessarily comes from a certain point A in a straight line to some point B. Consequently, each vector has not only a numerical value - length, but also a physical and geometric value - direction. From this comes the first, simplest definition of a vector. So, a vector is a directed segment coming from a point A to the point B. It is designated as follows: .
And to begin various operations with vectors , we need to get acquainted with one more definition of a vector.
A vector is a type of representation of a point that needs to be reached from some starting point. For example, a three-dimensional vector is usually written as (x, y, z) . In very simple terms, these numbers mean how far you need to walk in three different directions to get to a point.
Let a vector be given. Wherein x = 3 (right hand points to the right), y = 1 (left hand points forward) z = 5 (under the point there is a staircase leading up). Using this data, you will find a point by walking 3 meters in the direction indicated by your right hand, then 1 meter in the direction indicated by your left hand, and then a ladder awaits you and, rising 5 meters, you will finally find yourself at the end point.
All other terms are clarifications of the explanation presented above, necessary for various operations on vectors, that is, solving practical problems. Let's go through these more rigorous definitions, focusing on typical vector problems.
Physical examples vector quantities can be the displacement of a material point moving in space, the speed and acceleration of this point, as well as the force acting on it.
Geometric vector presented in two-dimensional and three-dimensional space in the form directional segment. This is a segment that has a beginning and an end.
If A- the beginning of the vector, and B- its end, then the vector is denoted by the symbol or one lowercase letter . In the figure, the end of the vector is indicated by an arrow (Fig. 1)
Length(or module) of a geometric vector is the length of the segment generating it
The two vectors are called equal , if they can be combined (if the directions coincide) by parallel transfer, i.e. if they are parallel, directed in the same direction and have equal lengths.
In physics it is often considered pinned vectors, specified by the point of application, length and direction. If the point of application of the vector does not matter, then it can be transferred, maintaining its length and direction, to any point in space. In this case, the vector is called free. We will agree to consider only free vectors.
Linear operations on geometric vectors
Multiplying a vector by a number
Product of a vector per number is a vector that is obtained from a vector by stretching (at ) or compressing (at ) by a factor, and the direction of the vector remains the same if , and changes to the opposite if . (Fig. 2)
From the definition it follows that the vectors and = are always located on one or parallel lines. Such vectors are called collinear. (We can also say that these vectors are parallel, but in vector algebra it is customary to say “collinear.”) The converse is also true: if the vectors are collinear, then they are related by the relation
Consequently, equality (1) expresses the condition of collinearity of two vectors.
Addition and subtraction of vectors
When adding vectors you need to know that amount vectors and is called a vector, the beginning of which coincides with the beginning of the vector, and the end - with the end of the vector, provided that the beginning of the vector is attached to the end of the vector. (Fig. 3)
This definition can be distributed over any finite number of vectors. Let them be given in space n free vectors. When adding several vectors, their sum is taken to be the closing vector, the beginning of which coincides with the beginning of the first vector, and the end with the end of the last vector. That is, if you attach the beginning of the vector to the end of the vector, and the beginning of the vector to the end of the vector, etc. and, finally, to the end of the vector - the beginning of the vector, then the sum of these vectors is the closing vector 
, the beginning of which coincides with the beginning of the first vector, and the end - with the end of the last vector. (Fig. 4)
The terms are called components of the vector, and the formulated rule is polygon rule. This polygon may not be flat.
When a vector is multiplied by the number -1, the opposite vector is obtained. The vectors and have the same lengths and opposite directions. Their sum gives zero vector, whose length is zero. The direction of the zero vector is not defined.
In vector algebra, there is no need to consider the subtraction operation separately: subtracting a vector from a vector means adding the opposite vector to the vector, i.e. 
Example 1. Simplify the expression:
.
,
that is, vectors can be added and multiplied by numbers in the same way as polynomials (in particular, also problems on simplifying expressions). Typically, the need to simplify linearly similar expressions with vectors arises before calculating the products of vectors.
Example 2. Vectors and serve as diagonals of the parallelogram ABCD (Fig. 4a). Express through and the vectors , , and , which are the sides of this parallelogram.
Solution. The point of intersection of the diagonals of a parallelogram bisects each diagonal. We find the lengths of the vectors required in the problem statement either as half the sums of the vectors that form a triangle with the required ones, or as half the differences (depending on the direction of the vector serving as the diagonal), or, as in the latter case, half the sum taken with a minus sign. The result is the vectors required in the problem statement:
There is every reason to believe that you have now correctly answered the question about the vectors “Entrepreneurship” and “Innovative abilities” at the beginning of this lesson. Correct answer: an addition operation is performed on these vectors.
Solve vector problems yourself and then look at the solutions
How to find the length of the sum of vectors?
This problem occupies a special place in operations with vectors, since it involves the use of trigonometric properties. Let's say you come across a task like the following:
The vector lengths are given 
and the length of the sum of these vectors. Find the length of the difference between these vectors.
Solutions to this and other similar problems and explanations of how to solve them are in the lesson " Vector addition: length of the sum of vectors and the cosine theorem ".
And you can check the solution to such problems at Online calculator "Unknown side of a triangle (vector addition and cosine theorem)" .
Where are the products of vectors?
Vector-vector products are not linear operations and are considered separately. And we have lessons "Scalar product of vectors" and "Vector and mixed products of vectors".
Projection of a vector onto an axis
The projection of a vector onto an axis is equal to the product of the length of the projected vector and the cosine of the angle between the vector and the axis:
As is known, the projection of a point A on the straight line (plane) is the base of the perpendicular dropped from this point onto the straight line (plane).
Let be an arbitrary vector (Fig. 5), and and be the projections of its origin (points A) and end (points B) per axis l. (To construct a projection of a point A) draw a straight line through the point A a plane perpendicular to a straight line. The intersection of the line and the plane will determine the required projection.
Vector component on the l axis is called such a vector lying on this axis, the beginning of which coincides with the projection of the beginning, and the end with the projection of the end of the vector.
Projection of the vector onto the axis l called number
,
equal to the length of the component vector on this axis, taken with a plus sign if the direction of the components coincides with the direction of the axis l, and with a minus sign if these directions are opposite.
Basic properties of vector projections onto an axis:
1. Projections of equal vectors onto the same axis are equal to each other.
2. When a vector is multiplied by a number, its projection is multiplied by the same number.
3. The projection of the sum of vectors onto any axis is equal to the sum of the projections of the summands of the vectors onto the same axis.
4. The projection of the vector onto the axis is equal to the product of the length of the projected vector and the cosine of the angle between the vector and the axis:
.
Solution. Let's project vectors onto the axis l as defined in the theoretical background above. From Fig. 5a it is obvious that the projection of the sum of vectors is equal to the sum of the projections of vectors. We calculate these projections:
We find the final projection of the sum of vectors:
Relationship between a vector and a rectangular Cartesian coordinate system in space
Getting to know rectangular Cartesian coordinate system in space took place in the corresponding lesson, it is advisable to open it in a new window.
In an ordered system of coordinate axes 0xyz axis Ox called x-axis, axis 0y – y-axis, and axis 0z – axis applicate.
With an arbitrary point M space connect vector
called radius vector points M and project it onto each of the coordinate axes. Let us denote the magnitudes of the corresponding projections:
Numbers x, y, z are called coordinates of point M, respectively abscissa, ordinate And applicate, and are written as an ordered point of numbers: M(x;y;z)(Fig. 6).
A vector of unit length whose direction coincides with the direction of the axis is called unit vector(or ortom) axes. Let us denote by
Accordingly, the unit vectors of the coordinate axes Ox, Oy, Oz
Theorem. Any vector can be expanded into unit vectors of coordinate axes:
(2)
Equality (2) is called the expansion of the vector along the coordinate axes. The coefficients of this expansion are the projections of the vector onto the coordinate axes. Thus, the coefficients of expansion (2) of the vector along the coordinate axes are the coordinates of the vector.
After choosing a certain coordinate system in space, the vector and the triplet of its coordinates uniquely determine each other, so the vector can be written in the form
Representations of the vector in the form (2) and (3) are identical.
Condition for collinearity of vectors in coordinates
As we have already noted, vectors are called collinear if they are related by the relation
Let the vectors be given 
. These vectors are collinear if the coordinates of the vectors are related by the relation
,
that is, the coordinates of the vectors are proportional.
Example 6. Vectors are given 
. Are these vectors collinear?
Solution. Let's find out the relationship between the coordinates of these vectors:
.
The coordinates of the vectors are proportional, therefore, the vectors are collinear, or, what is the same, parallel.
Vector length and direction cosines
Due to the mutual perpendicularity of the coordinate axes, the length of the vector
equal to the length of the diagonal of a rectangular parallelepiped built on vectors
and is expressed by the equality
(4)
A vector is completely defined by specifying two points (start and end), so the coordinates of the vector can be expressed in terms of the coordinates of these points.
Let, in a given coordinate system, the origin of the vector be at the point
and the end is at the point
From equality
Follows that
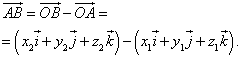
or in coordinate form
Hence, vector coordinates are equal to the differences between the same coordinates of the end and beginning of the vector . Formula (4) in this case will take the form
The direction of the vector is determined direction cosines . These are the cosines of the angles that the vector makes with the axes Ox, Oy And Oz. Let us denote these angles accordingly α , β And γ . Then the cosines of these angles can be found using the formulas
The direction cosines of a vector are also the coordinates of the vector of that vector and thus the vector of the vector
.
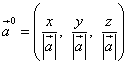
Considering that the length of the unit vector is equal to one unit, that is
,
we obtain the following equality for the direction cosines:
Example 7. Find the length of the vector x = (3; 0; 4).
Solution. The length of the vector is
Example 8. Points given:
Find out whether the triangle constructed on these points is isosceles.
Solution. Using the vector length formula (6), we find the lengths of the sides and determine whether there are two equal ones among them:
Two equal sides have been found, therefore there is no need to look for the length of the third side, and the given triangle is isosceles.
Example 9. Find the length of the vector and its direction cosines if 
.
Solution. The vector coordinates are given:
.
The length of the vector is equal to the square root of the sum of the squares of the vector coordinates:
.
Finding direction cosines:
Solve the vector problem yourself, and then look at the solution
Operations on vectors given in coordinate form
Let two vectors and be given, defined by their projections:
Let us indicate actions on these vectors.
Unit vector- This vector, the absolute value (modulus) of which is equal to unity. To denote a unit vector, we will use the subscript e. So, if a vector is given A, then its unit vector will be the vector A e. This unit vector is directed in the same direction as the vector itself A, and its module is equal to one, that is, a e = 1.
Obviously, A= a A e (a - vector module A). This follows from the rule by which the operation of multiplying a scalar by a vector is performed.
Unit vectors often associated with the coordinate axes of a coordinate system (in particular, with the axes of a Cartesian coordinate system). The directions of these vectors coincide with the directions of the corresponding axes, and their origins are often combined with the origin of the coordinate system.
Let me remind you that Cartesian coordinate system in space, a trio of mutually perpendicular axes intersecting at a point called the origin of coordinates is traditionally called. Coordinate axes are usually denoted by the letters X, Y, Z and are called the abscissa axis, ordinate axis and applicate axis, respectively. Descartes himself used only one axis, on which abscissas were plotted. Merit of use systems axes belongs to his students. Therefore the phrase Cartesian coordinate system historically wrong. It's better to talk rectangular coordinate system or orthogonal coordinate system. However, we will not change traditions and in the future we will assume that Cartesian and rectangular (orthogonal) coordinate systems are one and the same.
Unit vector, directed along the X axis, is denoted i, unit vector, directed along the Y axis, is denoted j, A unit vector, directed along the Z axis, is denoted k. Vectors i, j, k are called orts(Fig. 12, left), they have single modules, that is
i = 1, j = 1, k = 1.
Axes and unit vectors rectangular coordinate system in some cases they have different names and designations. Thus, the abscissa axis X can be called the tangent axis, and its unit vector is denoted τ (Greek small letter tau), the ordinate axis is the normal axis, its unit vector is denoted n, the applicate axis is the binormal axis, its unit vector is denoted b. Why change names if the essence remains the same?
The fact is that, for example, in mechanics, when studying the movement of bodies, the rectangular coordinate system is used very often. So, if the coordinate system itself is stationary, and the change in the coordinates of a moving object is tracked in this stationary system, then usually the axes are designated X, Y, Z, and their unit vectors respectively i, j, k.
But often, when an object moves along some kind of curvilinear path (for example, in a circle), it is more convenient to consider mechanical processes in the coordinate system moving with this object. It is for such a moving coordinate system that other names of axes and their unit vectors are used. It's just the way it is. In this case, the X axis is directed tangentially to the trajectory at the point where this object is currently located. And then this axis is no longer called the X axis, but the tangent axis, and its unit vector is no longer designated i, A τ . The Y axis is directed along the radius of curvature of the trajectory (in the case of motion in a circle - to the center of the circle). And since the radius is perpendicular to the tangent, the axis is called the normal axis (perpendicular and normal are the same thing). The unit vector of this axis is no longer denoted j, A n. The third axis (formerly Z) is perpendicular to the previous two. This is a binormal with an orth b(Fig. 12, right). By the way, in this case such rectangular coordinate system often referred to as "natural" or natural.
In geometry, a vector is a directed segment or an ordered pair of points in Euclidean space. Ortom vector is the unit vector of a normalized vector space or a vector whose norm (length) is equal to one.
You will need
- Knowledge of geometry.
Instructions
First you need to calculate the length vector. As is known, length (modulus) vector equal to the square root of the sum of the squares of the coordinates. Let a vector with coordinates: a(3, 4) be given. Then its length is |a| = (9 + 16)^1/2 or |a|=5.
To find the ort vector a, you need to divide each one by its length. The result will be a vector called an orth or unit vector. For vector a(3, 4) ort will be the vector a(3/5, 4/5). Vector a` will be unit for vector A.
To check whether the ort is found correctly, you can do the following: find the length of the resulting ort; if it is equal to one, then everything was found correctly; if not, then an error has crept into the calculations. Let's check whether the ort a` is found correctly. Length vector a` is equal to: a` = (9/25 + 16/25)^1/2 = (25/25)^1/2 = 1. So, the length vector a` is equal to one, which means the unit vector was found correctly.
Or the unit vector (unit vector of a normalized vector space) is a vector whose norm (length) is equal to one. Unit vector ... Wikipedia
- (ort) vector, the length of which is equal to the unit of the selected scale... Big Encyclopedic Dictionary
- (ort), a vector whose length is equal to the unit of the selected scale. * * * UNIT VECTOR UNIT VECTOR (ort), a vector whose length is equal to the unit of the selected scale... encyclopedic Dictionary
Ort, a vector whose length is equal to the unit of the selected scale. Any vector a can be obtained from some E.v. collinear to it. e by multiplying by the number (scalar) λ, i.e. a = λe. See also Vector calculus... Great Soviet Encyclopedia
- (ort), vector, the length of which is equal to the unit of the selected scale... Natural science. encyclopedic Dictionary
Orth: Wiktionary has an article “orth” Orth, or Orth the two-headed dog, the offspring of Typhon and Echidna, brother of Cerberus. Ort ... Wikipedia
A; m. [German] Ort] 1. Horn. A horizontal underground mine opening that does not have direct access to the surface. 2. Math. A vector whose length is equal to one. * * * unit vector I (from the Greek orthós straight), the same as the unit vector. II (German... ... encyclopedic Dictionary
The change in coordinates x2 - x1 is usually denoted by the symbol Δx12 (read “delta x one, two”). This entry means that during the period of time from moment t1 to moment t2 the change in the coordinate of the body is Δx12 = x2 - x1. Thus, if the body moved in the positive direction of the X axis of the selected coordinate system (x2 > x1), then Δx12 >
In Fig. 45 shows a point body B, which moves in the negative direction of the X axis. Over the period of time from t1 to t2, it moves from a point with a larger coordinate x1 to a point with a smaller coordinate x2. As a result, the change in the coordinate of point B over the considered period of time is Δx12 = x2 - x1 = (2 - 5) m = -3 m. The displacement vector in this case will be directed in the negative direction of the X axis, and its module |Δx12| equal to 3 m. From the examples considered, the following conclusions can be drawn.
In the examples considered (see Fig. 44 and 45), the body was always moving in one direction.
How to find the displacement module in physics? (Maybe there is some universal formula?)
Therefore, the path traveled by it is equal to the modulus of change in the body’s coordinates and the modulus of displacement: s12 = |Δx12|.
Let us determine the change in coordinates and displacement of the body over the time period from t0 = 0 to t2 = 7 s. In accordance with the definition, change in coordinate Δx02 = x2 - x0 = 2 m >
Now let’s determine the path that the body has traveled in the same period of time from t0 = 0 to t2 = 7 s. First, the body traveled 8 m in one direction (which corresponds to the modulus of coordinate change Δx01), and then 6 m in the opposite direction (this value corresponds to the modulus of coordinate change Δx12). This means that the whole body traveled 8 + 6 = 14 (m). By definition of the path, during the time interval from t0 to t2 the body traveled a distance s02 = 14 m.
Results
The movement of a point over a period of time is a directed segment of a straight line, the beginning of which coincides with the initial position of the point, and the end with the final position of the point.
Questions
Exercises
Vectors, actions with vectors
Pythagorean theorem cosine theorem
We will denote the length of the vector by . The modulus of a number has a similar notation, and the length of a vector is often called the modulus of a vector.
, where 
.
Thus, 
.
Let's look at an example.
:
.
Thus, vector length 
.
Calculate Vector Length 
, hence, 
Top of page
Let's look at the solutions to the examples.
.
Moving
:
:
.
.
Top of page
Thus, .
or ,or ,
No time to figure it out?
Order a solution
Top of page
Until now, we have considered only rectilinear uniform motion. In this case, the point bodies moved in the selected reference system either in the positive or negative direction of the X coordinate axis. We found that depending on the direction of movement of the body, for example, during the period of time from moment t1 to moment t2, the change in the coordinate of the body (x2 - x1 ) can be positive, negative or equal to zero (if x2 = x1).
The change in coordinates x2 - x1 is usually denoted by the symbol Δx12 (read “delta x one, two”). This entry means that during the period of time from moment t1 to moment t2 the change in the coordinate of the body is Δx12 = x2 - x1. Thus, if the body moved in the positive direction of the X axis of the selected coordinate system (x2 > x1), then Δx12 > 0. If the movement occurred in the negative direction of the X axis (x21), then Δx12
It is convenient to determine the result of the movement using a vector quantity. Such a vector quantity is displacement.
The movement of a point over a period of time is a directed segment of a straight line, the beginning of which coincides with the initial position of the point, and the end with the final position of the point.
Like any vector quantity, displacement is characterized by modulus and direction.
We will record the vector of movement of a point over the period of time from t1 to t2 in the following way: Δx12.
Let us explain this with an example. Let some point A (point body) move in the positive direction of the X axis and, over a period of time from t1 to t2, move from a point with coordinate x1 to a point with a larger coordinate x2 (Fig. 44). In this case, the displacement vector is directed in the positive direction of the X axis, and its magnitude is equal to the change in coordinate over the period of time under consideration: Δx12 = x2 - x1 = (5 - 2) m = 3 m.
In Fig. 45 shows a point body B, which moves in the negative direction of the X axis.
Over the period of time from t1 to t2, it moves from a point with a larger coordinate x1 to a point with a smaller coordinate x2. As a result, the change in the coordinate of point B over the considered period of time is Δx12 = x2 - x1 = (2 - 5) m = -3 m. The displacement vector in this case will be directed in the negative direction of the X axis, and its module |Δx12| equal to 3 m. From the examples considered, the following conclusions can be drawn.
The direction of movement during rectilinear movement in one direction coincides with the direction of movement.
The modulus of the displacement vector is equal to the modulus of the change in the coordinates of the body over the considered period of time.
In everyday life, the concept of “path” is used to describe the final result of movement. Usually the path is denoted by the symbol S.
The path is the entire distance traveled by a point body during the period of time under consideration.
Like any distance, path is a non-negative quantity. For example, the path traveled by point A in the example considered (see Fig. 44) is equal to three meters. The distance traveled by point B is also three meters.
In the examples considered (see Fig. 44 and 45), the body was always moving in one direction. Therefore, the path traveled by it is equal to the modulus of change in the body’s coordinates and the modulus of displacement: s12 = |Δx12|.
If the body moved all the time in one direction, then the path traveled by it is equal to the displacement module and the coordinate change module.
The situation will change if the body changes direction of movement during the period of time under consideration.
In Fig. 46 shows how a point body moved from the moment t0 = 0 to the moment t2 = 7 s. Until the moment t1 = 4 s, the movement occurred uniformly in the positive direction of the X axis. As a result, the change in coordinates Δx01 = x1 - x0 = (11 - 3) m = -8 m. After this, the body began to move in the negative direction of the X axis until the moment t2 = 7 s. In this case, the change in its coordinates is Δx12 = x2 - x1 = (5 - 11) m = -6 m. The graph of this movement is shown in Fig. 47.
Let us determine the change in coordinates and displacement of the body over the time period from t0 = 0 to t2 = 7 s. In accordance with the definition, the change in coordinate Δx02 = x2 - x0 = 2 m > 0. Therefore, the displacement Δx02 is directed in the positive direction of the X axis, and its module is equal to 2 m.
Now let’s determine the path that the body has traveled in the same period of time from t0 = 0 to t2 = 7 s. First, the body traveled 8 m in one direction (which corresponds to the modulus of coordinate change Δx01), and then 6 m in the opposite direction (this value corresponds to the modulus of coordinate change Δx12).
Trajectory
This means that the whole body traveled 8 + 6 = 14 (m). By definition of the path, during the time interval from t0 to t2 the body traveled a distance s02 = 14 m.
The analyzed example allows us to conclude:
In the case when a body changes the direction of its movement during the considered period of time, the path (the entire distance traveled by the body) is greater than both the modulus of displacement of the body and the modulus of change in the coordinates of the body.
Now imagine that the body, after time t2 = 7 s, continued its movement in the negative direction of the X axis until t3 = 8 s in accordance with the law shown in Fig. 47 dotted line. As a result, at the moment of time t3 = 8 s, the coordinate of the body became equal to x3 = 3 m. It is easy to determine that in this case the movement of the body over the period of time from t0 to t3 s is equal to Δx13 = 0.
It is clear that if we only know the displacement of a body during its movement, then we cannot say how the body moved during this time. For example, if it was only known about a body that its initial and final coordinates are equal, then we would say that during the movement the displacement of this body is zero. It would be impossible to say anything more specific about the nature of the movement of this body. Under such conditions, the body could generally stand still for the entire period of time.
The movement of a body over a certain period of time depends only on the initial and final coordinates of the body and does not depend on how the body moved during this period of time.
Results
The movement of a point over a period of time is a directed segment of a straight line, the beginning of which coincides with the initial position of the point, and the end with the final position of the point.
The movement of a point body is determined only by the final and initial coordinates of the body and does not depend on how the body moved during the considered period of time.
Path is the entire distance traveled by a point body during the period of time under consideration.
If the body did not change the direction of movement during the movement, then the path traveled by this body is equal to the modulus of its displacement.
If the body changed the direction of its movement during the considered period of time, the path is greater than both the modulus of displacement of the body and the modulus of change in the coordinates of the body.
The path is always a non-negative quantity. It is equal to zero only if during the entire period of time under consideration the body was at rest (standing still).
Questions
- What is movement? What does it depend on?
- What is a path? What does it depend on?
- How does a path differ from moving and changing coordinates over the same period of time, during which the body moved in a straight line without changing the direction of movement?
Exercises
- Using the law of motion in graphical form, presented in Fig. 47, describe the nature of the body’s movement (direction, speed) at different time intervals: from t0 to t1, from t1 to t2, from t2 to t3.
- The dog Proton ran out of the house at time t0 = 0, and then, at the command of its owner, at time t4 = 4 s, rushed back. Knowing that Proton was running in a straight line all the time and its velocity magnitude |v| = 4 m/s, determine graphically: a) the change in the coordinates and path of the Proton over the period of time from t0 = 0 to t6 = 6 s; b) the path of the Proton over the time interval from t2 = 2 s to t5 = 5 s.
Vectors, actions with vectors
Finding the length of a vector, examples and solutions.
By definition, a vector is a directed segment, and the length of this segment on a given scale is the length of the vector. Thus, the task of finding the length of a vector on the plane and in space is reduced to finding the length of the corresponding segment. To solve this problem we have at our disposal all the means of geometry, although in most cases it is sufficient Pythagorean theorem. With its help, you can obtain a formula for calculating the length of a vector from its coordinates in a rectangular coordinate system, as well as a formula for finding the length of a vector from the coordinates of its start and end points. When the vector is a side of a triangle, its length can be found by cosine theorem, if the lengths of the other two sides and the angle between them are known.
Finding the length of a vector from coordinates.
We will denote the length of the vector by .
physical dictionary (kinematics)
The modulus of a number has a similar notation, and the length of a vector is often called the modulus of a vector.
Let's start by finding the length of a vector on a plane using coordinates.
Let us introduce a rectangular Cartesian coordinate system Oxy on the plane. Let a vector be specified in it and have coordinates . We obtain a formula that allows us to find the length of a vector through the coordinates and .
Let us plot the vector from the origin (from point O). Let us denote the projections of point A onto the coordinate axes as and, respectively, and consider a rectangle with diagonal OA.
By virtue of the Pythagorean theorem, the equality , where . From the definition of vector coordinates in a rectangular coordinate system, we can assert that and , and by construction, the length OA is equal to the length of the vector, therefore, .
Thus, formula for finding the length of a vector according to its coordinates on the plane has the form .
If the vector is represented as a decomposition in coordinate vectors , then its length is calculated using the same formula , since in this case the coefficients and are the coordinates of the vector in a given coordinate system.
Let's look at an example.
Find the length of the vector given in the Cartesian coordinate system.
We immediately apply the formula to find the length of the vector from the coordinates :
Now we get the formula for finding the length of the vector according to its coordinates in the rectangular Oxyz coordinate system in space.
Let us plot the vector from the origin and denote the projections of point A onto the coordinate axes as and . Then we can build a rectangular parallelepiped on the sides, in which OA will be the diagonal.
In this case (since OA is the diagonal of a rectangular parallelepiped), whence . Determining the coordinates of the vector allows us to write equalities , and the length OA is equal to the desired length of the vector, therefore, .
Thus, vector length in space is equal to the square root of the sum of the squares of its coordinates, that is, found by the formula .
Calculate Vector Length , where are the unit vectors of the rectangular coordinate system.
We are given a vector decomposition into coordinate vectors of the form , hence, . Then, using the formula for finding the length of a vector from coordinates, we have .
Top of page
The length of a vector through the coordinates of its start and end points.
How to find the length of a vector if the coordinates of its beginning and end points are given?
In the previous paragraph, we obtained formulas for finding the length of a vector from its coordinates on a plane and in three-dimensional space. Then we can use them if we find the coordinates of the vector from the coordinates of the points of its beginning and end.
Thus, if points and are given on the plane, then the vector has coordinates and its length is calculated by the formula , and the formula for finding the length of a vector from the coordinates of points and three-dimensional space has the form .
Let's look at the solutions to the examples.
Find the length of the vector if in a rectangular Cartesian coordinate system .
You can immediately apply the formula to find the length of a vector from the coordinates of the start and end points on the plane :
The second solution is to determine the coordinates of the vector through the coordinates of the points and apply the formula :
.
Determine at what values the length of the vector is equal if .
The length of the vector from the coordinates of the start and end points can be found as
Equating the resulting value of the vector length to , we calculate the required ones:
Top of page
Finding the length of a vector using the cosine theorem.
Most problems involving finding the length of a vector are solved in coordinates. However, when the coordinates of the vector are not known, we have to look for other solutions.
Let the lengths of two vectors and the angle between them (or the cosine of the angle) be known, and you need to find the length of the vector or . In this case, using the cosine theorem in triangle ABC, you can calculate the length of side BC, which is equal to the desired length of the vector.
Let's analyze the solution of the example to clarify what has been said.
The lengths of the vectors and are equal to 3 and 7, respectively, and the angle between them is equal to . Calculate the length of the vector.
The length of the vector is equal to the length of side BC in triangle ABC. From the condition we know the lengths of the sides AB and AC of this triangle (they are equal to the lengths of the corresponding vectors), as well as the angle between them, so we have enough data to apply the cosine theorem:
Thus, .
So, to find the length of a vector from coordinates, we use the formulas or ,
according to the coordinates of the start and end points of the vector - or ,
in some cases the cosine theorem leads to the result.
No time to figure it out?
Order a solution
Top of page
- Bugrov Ya.S., Nikolsky S.M. Higher mathematics. Volume one: elements of linear algebra and analytical geometry.
- Atanasyan L.S., Butuzov V.F., Kadomtsev S.B., Poznyak E.G., Yudina I.I. Geometry. Grades 7 – 9: textbook for general education institutions.
- Atanasyan L.S., Butuzov V.F., Kadomtsev S.B., Kiseleva L.S., Poznyak E.G. Geometry. Textbook for 10-11 grades of secondary school.
Search Lectures
Scalar square vector
What happens if a vector is multiplied by itself?
The number is called scalar square vector, and are denoted as .
Thus, scalar square vectorequal to the square of the length of a given vector: